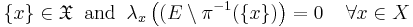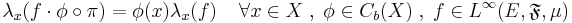Lifting theory
Lifting theory was first introduced in a series of pioneering papers by John von Neumann.[1] A lifting on a measure space 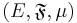 is a linear and multiplicative inverse
is a linear and multiplicative inverse
of the quotient map
In other words, a lifting picks from every equivalence class ![\underline{}[f]](/2012-wikipedia_en_all_nopic_01_2012/I/54de4f2dc7aa4e37b46792250a3122be.png) of bounded measurable functions modulo negligible functions a representative
of bounded measurable functions modulo negligible functions a representative
— which is henceforth written ![\underline{}T([f])](/2012-wikipedia_en_all_nopic_01_2012/I/58363571fb171f84837bd875306889a4.png) or
or ![\underline{}T[f]](/2012-wikipedia_en_all_nopic_01_2012/I/b55d7f777de05baae77b9dbed6e83871.png) or simply
or simply 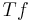 —
—
in such a way that
Liftings are used to produce disintegrations of measures, for instance conditional probability distributions given continuous random variables, and fibrations of Lebesgue measure on the level sets of a function.
Contents |
Existence of liftings
Theorem. Suppose 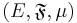 is complete.[2] Then
is complete.[2] Then 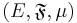 admits a lifting if and only if there exists a collection of mutually disjoint integrable sets in
admits a lifting if and only if there exists a collection of mutually disjoint integrable sets in 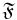 whose union is
whose union is  .
.
In particular, if 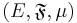 is the completion of a sigma-finite[3] measure or of an inner regular Borel measure on a locally compact space, then
is the completion of a sigma-finite[3] measure or of an inner regular Borel measure on a locally compact space, then 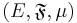 admits a lifting.
admits a lifting.
The proof consists in extending a lifting to ever larger sub-sigma-algebras, applying Doob's martingale convergence theorem if one encounters a countable chain in the process.
Strong liftings
Suppose now that 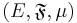 is complete and that
is complete and that 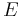 comes equipped with a completely regular Hausdorff topology
comes equipped with a completely regular Hausdorff topology 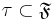 such that the union of any collection of negligible open sets is again negligible – this is the case if
such that the union of any collection of negligible open sets is again negligible – this is the case if 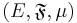 is sigma-finite or comes from a Radon measure. Then the support of
is sigma-finite or comes from a Radon measure. Then the support of  ,
, 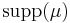 , can be defined as the complement of the largest negligible open subset, and the collection
, can be defined as the complement of the largest negligible open subset, and the collection 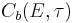 of bounded continuous functions belongs to
of bounded continuous functions belongs to 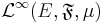 .
.
A strong lifting for 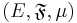 is a lifting
is a lifting 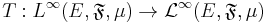 such that
such that 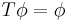 on
on 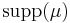 for all
for all 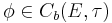 . This is the same as requiring that[4]
. This is the same as requiring that[4] 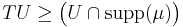 for all open sets
for all open sets 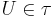 .
.
Theorem. If 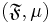 is sigma-finite and complete and
is sigma-finite and complete and 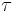 has a countable basis then
has a countable basis then 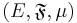 admits a strong lifting.
admits a strong lifting.
Proof. Let  be a lifting for
be a lifting for 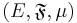 and
and 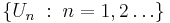 a countable basis for
a countable basis for 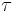 . For any point
. For any point  in the negligible set
in the negligible set 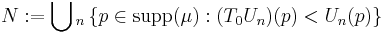 let
let  be any character[5] on
be any character[5] on  that extends the character
that extends the character  of
of 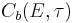 . Then define, for
. Then define, for 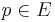 and
and ![[f]\in L^\infty](/2012-wikipedia_en_all_nopic_01_2012/I/0b9f0b38efce28a0d563fd1e63a2560b.png) ,
,
 is the desired strong lifting.
is the desired strong lifting.
Application: disintegration of a measure
Suppose 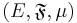 and
and 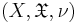 are sigma-finite measure spaces (
are sigma-finite measure spaces (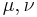 positive) and
positive) and 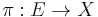 is a measurable map. A disintegration of
is a measurable map. A disintegration of 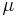 along
along 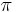 with respect to
with respect to 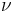 is a slew
is a slew 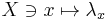 of positive sigma-additive measures on
of positive sigma-additive measures on 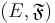 such that (1)
such that (1) 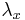 is carried by the fiber
is carried by the fiber 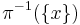 of
of 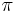 over
over 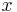 :
:
and (2) for every  -integrable function
-integrable function 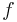 ,
,
-
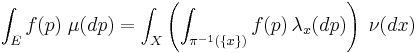 (*)
(*)
in the sense that, for  -almost all
-almost all 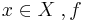 is
is 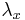 -integrable, the function
-integrable, the function 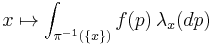 is
is  -integrable, and the displayed equality (*) obtains.
-integrable, and the displayed equality (*) obtains.
Disintegrations exist in various circumstances, the proofs varying but almost all using strong liftings. Here is a rather general result. Its short proof gives the general flavor.
Theorem. Suppose  is a polish[6] space and
is a polish[6] space and  a separable Hausdorff space, both equipped with their Borel sigma-algebras. Let
a separable Hausdorff space, both equipped with their Borel sigma-algebras. Let  be a sigma-finite Borel measure on
be a sigma-finite Borel measure on  and
and 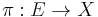 an
an 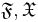 –measurable map. Then there exists a sigma-finite Borel measure
–measurable map. Then there exists a sigma-finite Borel measure  on
on  and a disintegration (*).
and a disintegration (*).
If  is finite,
is finite,  can be taken to be the pushforward[7]
can be taken to be the pushforward[7] 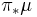 , and then the
, and then the 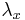 are probabilities.
are probabilities.
Proof. Because of the polish nature of  there is a sequence of compact subsets of
there is a sequence of compact subsets of  that are mutually disjoint, whose union has negligible complement, and on which
that are mutually disjoint, whose union has negligible complement, and on which 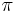 is continuous. This observation reduces the problem to the case that both
is continuous. This observation reduces the problem to the case that both  and
and  are compact and
are compact and 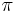 is continuous, and
is continuous, and 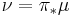 . Complete
. Complete 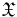 under
under  and fix a strong lifting
and fix a strong lifting  for
for 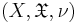 . Given a bounded
. Given a bounded  -measurable function
-measurable function 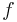 , let
, let 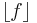 denote its conditional expectation under
denote its conditional expectation under  , i.e., the Radon-Nikodym derivative of[8]
, i.e., the Radon-Nikodym derivative of[8] 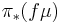 with respect to
with respect to 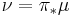 . Then set, for every
. Then set, for every 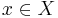 ,
, 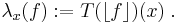 To show that this defines a disintegration is a matter of bookkeeping and a suitable Fubini theorem. To see how the strongness of the lifting enters, note that
To show that this defines a disintegration is a matter of bookkeeping and a suitable Fubini theorem. To see how the strongness of the lifting enters, note that
and take the infimum over all positive 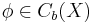 with
with  ; it becomes apparent that the support of
; it becomes apparent that the support of 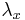 lies in the fiber over
lies in the fiber over  .
.
References
- Bellow, Alexandra (1969), Topics in the theory of lifting, Springer, Berlin, Heidelberg, New York
- ^ Bellow, Alexandra (1969), Topics in the theory of lifting, Springer, Berlin, Heidelberg, New York
- ^ A subset
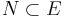 is locally negligible if it intersects every integrable set in
is locally negligible if it intersects every integrable set in 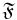 in a subset of a negligible set of
in a subset of a negligible set of 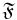 .
. 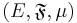 is complete if every locally negligible set is negligible and belongs to
is complete if every locally negligible set is negligible and belongs to 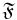 .
. - ^ i.e., there exists a countable collection of integrable sets – sets of finite measure in
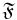 – that covers the underlying set
– that covers the underlying set  .
. - ^
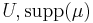 are identified with their indicator functions.
are identified with their indicator functions. - ^ A character on a unital algebra is a multiplicative linear functional with values in the coefficient field that maps the unit to 1.
- ^ A separable space is polish if its topology comes from a complete metric. In the present situation it would be sufficient to require that
 is Suslin, i.e., is the continuous Hausdorff image of a polish space.
is Suslin, i.e., is the continuous Hausdorff image of a polish space. - ^ The pushforward
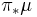 of
of  under
under  , also called the image of
, also called the image of  under
under  and denoted
and denoted 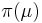 , is the measure
, is the measure  on
on 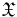 defined by
defined by 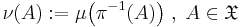 .
. - ^
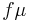 is the measure that has density
is the measure that has density 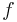 with respect to
with respect to 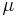
![[\ ]:\mathcal L^\infty(E,\mathfrak F,\mu)\to L^\infty(E,\mathfrak F,\mu)\;\;,\;
f\mapsto [f].\;](/2012-wikipedia_en_all_nopic_01_2012/I/e21876579ac15899e2cbcefd406c77d1.png)
![T(r[f]%2Bs[g])(p)=rT[f](p) %2B sT[g](p)\;\;\forall p\in E\;,r,s\in \mathbf R\;,](/2012-wikipedia_en_all_nopic_01_2012/I/45d1a03dc33b42546a48b2e5dff556a5.png)
![T([f]\times[g])(p)=T[f](p)\times T[g](p)\;\;\forall p\in E\;;](/2012-wikipedia_en_all_nopic_01_2012/I/d891b81b10b76d0437196b70ab793e72.png)
![\mathrm{one\ requires\ also\ that\ }T[1]=1\;.](/2012-wikipedia_en_all_nopic_01_2012/I/acde6d9f27d261c57160897218a2a4cf.png)
![\big(T[f]\big)(p):=\left\{
\begin{array}{lr}
\big(T_0[f]\big)(p)&\mathrm{\ if\ }p\notin N\\
\;\;\;\;T_p[f]&\mathrm{\ \ \ if\ }p\in N\;.\\
\end{array}
\right.](/2012-wikipedia_en_all_nopic_01_2012/I/62c89a7bb5e6b9a367e2cc376d57f560.png)